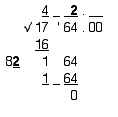How to Calculate the Square Root of Any Number by Hand
Created | Updated Sep 14, 2010
Calculating square roots by hand is not even what a practising mathematician would call a leisurely pastime. The procedure is hardly, if ever, taught in school in this age of computers. It is, however, quite possible to determine the square root of a number to any degree of accuracy for which a person is willing to stretch the work, and the mathematics involved in the procedure is not complex. A brief glimpse of a hand-calculated square root problem appears very much like a long division problem, and some aspects of the procedure are similar.
Step One - The Set-up
To illustrate these steps, we will find the square root of 1,234.56.
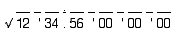
Write the number for which the square root is to be calculated under a square root sign (√), elongated if desired to simulate the appearance of long division. Locate and mark the decimal point of this number. Above the radical sign1, place another decimal point directly above the decimal point of the original number. This second point will become the decimal point in the square root of the original number. Note that since the handwritten work will look very similar to a long division problem, care should be given in allowing for space to write products, take differences, and carry down digits just as in long division.
On both sides of the decimal point in the original number, use some sort of marking system to pair off adjacent digits of the number. On the whole number side (the left side of the decimal point), cease pairing off digits when there are no digits or one digit left at the front of the number. On the fractional side (the right side of the decimal point), pair off digits using zeroes as placeholders if necessary. The digits in our example are paired off, with apostrophes as separators.
Each pairing of digits on the fractional (right) side will constitute one digit of accuracy for the final square root calculation, so if exceptional accuracy is desired, leave plenty of room on the right side of the paper for additional work. In the above example as it is presented, one would be able to calculate the square root of 1234.56 to four decimal places beyond the decimal point2. As many additional pairs of zeroes could be added to the end of the number as one wished to calculate.
Step Two - First Calculation
The calculation begins. Look at the very first pairing at the front of the number, similar in a sense to the way one looks at a number when beginning long division. This first pairing will either consist of one or two digits - for procedural purposes, it makes no difference. In our example, the first pairing is '12'. Determine the largest perfect square number (1, 4, 9, 16, 25, 36, 49, 64, and 81 will be your only choices for this first calculation) which is less than or equal to the value of the first pairing. In this case, the largest perfect square less than 12 is 9. Write this square number underneath the first pairing in preparation for subtraction, and write the square root of this perfect square above the radical sign, directly above the first pairing.

Subtract the perfect square from the value of the first pairing and write the difference below (12 - 9 = 3). Carry the next pairing of digits in the original number (34) down to the difference that was just written, and let the number comprising all these digits (334) be called D, for the sake of later referral.

So far, the look of the problem should seem very much like a long division problem as promised. It helps to conform to this style of presentation, since keeping track of differences and products will be as vital here as in division.
Step Three - The Trial-by-Error Repeating Step
The mathematics involved thus far has been relatively simple. The first spot of good news is that it only gets slightly more difficult in this third step. The second spot of good news is that with this somewhat more lengthy step, the procedure will begin to repeat indefinitely, until the degree of accuracy desired is finally obtained.
Multiply the number above the square root sign by 2, treating it as a whole number. (Ignore where the decimal might reside.) In our example, we have 3 above the radical, so we multiply it by 2 to obtain 6. Never mind that the decimal has not yet been reached. Place this product to the left of the number D. Leave space at the end of this product for one additional digit - our number is 6, so we write '6_'.

A digit between 0 and 9 must be chosen, at random if one wishes. Let's call it X. This digit will be written in 2 places: once above the square root sign, directly over the pairing that was just carried down (above the 34), and once in the blank space at the end of the product that was just calculated (after the 6). Let the letter Y denote the number thus created. In our example, if '7' is chosen for X, then Y will be 67.
Multiply X and Y. What is being sought is the largest possible value of X for which the product of X and Y will still be less than D. Choosing X to be as large as possible is purely a matter of trial and error. A glimmer of hope: there are only 10 choices for X, so hang in there! We can see that 7 is too big, because 7 * 67 = 469, which is greater than D (334). A little experimentation will show that the largest X we can choose is 5, making Y=65. (5 * 65 = 325 < 334)
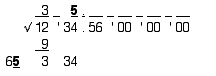
Once the correct value of X is found and the product of X and Y is calculated, this product is written underneath D, and subtracted to obtain a new difference. Then next pair of digits from the original number are then carried down to obtain a new D.

Now Step Three repeats, until the degree of accuracy desired is obtained. To continue our example, the number we write to the left of the new D is 35 * 2, which equals 70. We leave a space at the end and write '70_'. The X that will fill in the blank is 1, because 1 * 701 < 956, while any other choice of X is too big. The product of 1 and 701 is written under D and subtracted, and another pairing is brought down to obtain the next D.
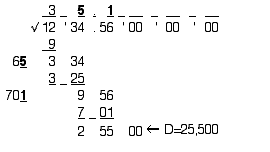
Worked out through all four decimal places, our example should look like this:

So, the square root of 1234.56, to four decimal places, is 35.1363. We can be sure about three of those decimal places, while the last '3' might round up, depending on the next digit.
More Examples
Here is a number (13,234.251) where the first pairing consists of a single digit.
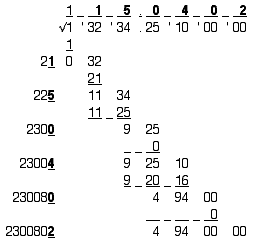
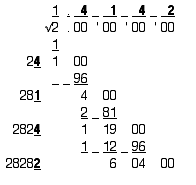
Here is a well-known square root:
Finally, an example that comes out to a whole number: