If you're looking for a challenging puzzle that can provide endless hours of gameplay, you might try your hand at pentominoes. It is based on a Roman game, but the term was coined by Solomon W Golomb in the 1950s¹. Pentominoes is somewhat a never-ending jigsaw puzzle with only 12 pieces that can be arranged in countless configurations and has thousands of possible solutions.

The Pieces

The pieces themselves are all the combinations you can make by aligning the edges of five squares². These resulting shapes will look familiar to anyone who has played Tetris³ as the same principle applies, with the only difference being that the pieces in Tetris are made up of four squares instead of five.

The game of pentominoes is described in Arthur C Clarke's book, Imperial Earth. In the book, the player is asked to first 'discover' all the pieces before continuing to the arrangements of the pieces. There are actually only 12 possible arrangements. Some people have adopted a shorthand alphabet for naming the pieces; they are named after the letters they most resemble. However, several of these take some imagination. F, I, L, N, P, T, U, V, W, X, Y and Z.

The Puzzle

The puzzle is played by arranging the pieces to fit an area of 60 squares. Actually this is inevitable since 12 pieces of five squares each will always take an area of 60 squares if they are not overlapping. However, rectangles are usually preferred. You can make 3x20, 4x15, 5x12 and 6x10 rectangles using all 12 pieces only once.

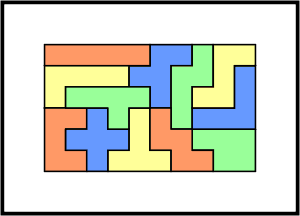
The most common arrangement is the 6x10 rectangle. There are 2,339 possible solutions to this, barring isomorphisms. This may look like an easy puzzle to solve, but it's not. Inevitably, what happens when you first try to solve pentominoes is that you tend to end up with the last piece and an empty hole that's the shape of a piece you've already used, not the shape of the piece you have in your hand. Quite frustrating and unfortunately it happens quite often. The other configurations have fewer possible solutions, but are easier to solve (probably for that very reason). For example, there are only two unique solutions to fit the pieces into an area of 3x20. Since it is such a long narrow shape, some of the pieces will only fit into it in certain orientations. Therefore, you don't need to try fitting a piece that is four squares on a side against a three square dimension of the rectangle.

Other configurations leave empty shapes in the centre (think of a square doughnut), or have internal angles (think of a large plus sign). But the stumper is still the 6 X 10 rectangle. All those possible solutions! And you might spend days or weeks before you find even a couple, or any at all for that matter.

Another common way to play pentominoes is called the replication problem (sometimes called the triplication problem). Try to recreate a scale model three times the dimensions of the original of each of the pieces using nine of the other pieces. This can be done with all 12 pentominoes.

The Game

Pentomino pieces can also be used in a competitive game for two or three players using a chess board with squares that conform to the size of the pieces. Each player in turn places a piece on the board. The pieces may not overlap or extend beyond the edges of the board. However, they don't need to be placed adjacently. The object of the game is to be the last player able to place a piece legally on the board. The game will last between five and 12 moves.

The pieces can be bought from online shops, or can be constructed at home using wooden craft blocks or even Lego bricks. Of course, if you have access to a wood shop, you could make a fine set by using a scroll saw and some nice hardwood. Or you could even use thin balsa and a sharp knife, but these do tend to get broken.

A very good source for more information on pentominoes and other two- and three-dimensional polyforms can be found at The Poly Pages.